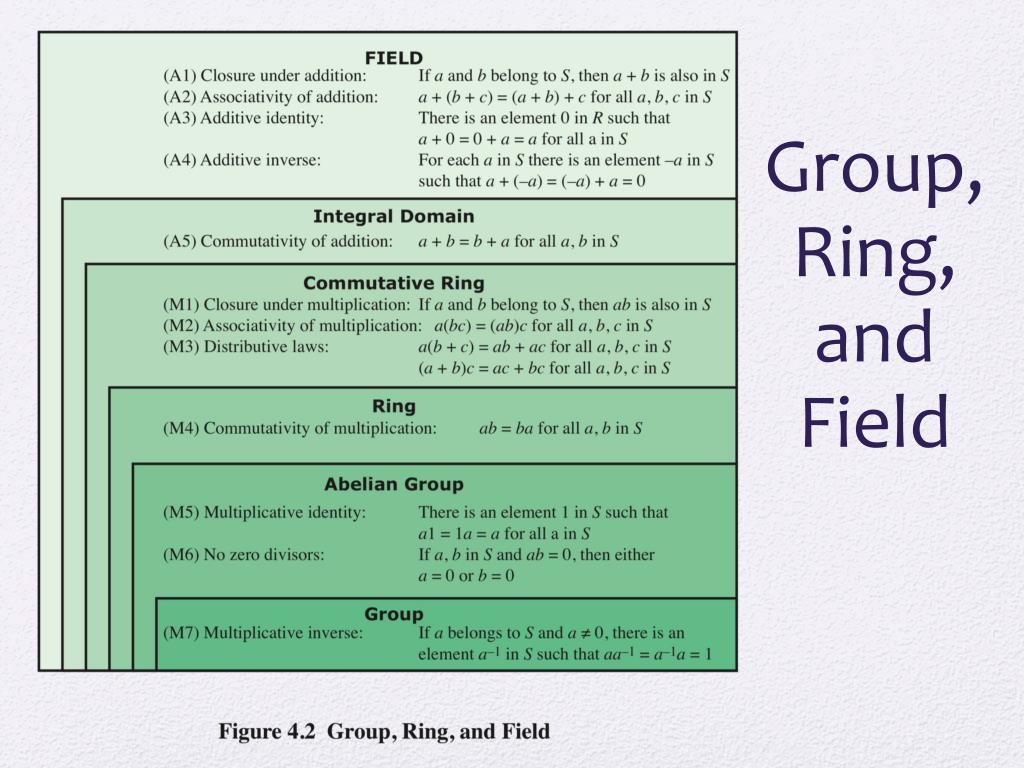
Field Definition In Ring Theory . fields are fundamental objects in number theory, algebraic geometry, and many other areas of mathematics. a field is a group under both addition and multiplication. ring theory studies the structure of rings, their representations, or, in different language, modules, special classes of. the structures similar to the set of integers are called rings, and those similar to the set of real numbers are. a field is a ring such that the second operation also satisfies all the properties of an abelian group (after throwing out the. A field is a commutative ring in which every nonzero element is a unit. A group is a set g which is closed under an operation ∗.
from exollekjz.blob.core.windows.net
a field is a group under both addition and multiplication. A field is a commutative ring in which every nonzero element is a unit. fields are fundamental objects in number theory, algebraic geometry, and many other areas of mathematics. a field is a ring such that the second operation also satisfies all the properties of an abelian group (after throwing out the. the structures similar to the set of integers are called rings, and those similar to the set of real numbers are. ring theory studies the structure of rings, their representations, or, in different language, modules, special classes of. A group is a set g which is closed under an operation ∗.
Ring Vs Field at Molly Nix blog
Field Definition In Ring Theory a field is a group under both addition and multiplication. fields are fundamental objects in number theory, algebraic geometry, and many other areas of mathematics. the structures similar to the set of integers are called rings, and those similar to the set of real numbers are. A group is a set g which is closed under an operation ∗. a field is a group under both addition and multiplication. a field is a ring such that the second operation also satisfies all the properties of an abelian group (after throwing out the. A field is a commutative ring in which every nonzero element is a unit. ring theory studies the structure of rings, their representations, or, in different language, modules, special classes of.
From www.slideserve.com
PPT Rings and fields PowerPoint Presentation, free download ID2872841 Field Definition In Ring Theory a field is a ring such that the second operation also satisfies all the properties of an abelian group (after throwing out the. a field is a group under both addition and multiplication. the structures similar to the set of integers are called rings, and those similar to the set of real numbers are. A field is. Field Definition In Ring Theory.
From www.youtube.com
Theorem on field/ring theory /Bs math semester vi YouTube Field Definition In Ring Theory the structures similar to the set of integers are called rings, and those similar to the set of real numbers are. fields are fundamental objects in number theory, algebraic geometry, and many other areas of mathematics. a field is a group under both addition and multiplication. ring theory studies the structure of rings, their representations, or,. Field Definition In Ring Theory.
From www.slideserve.com
PPT Rings,Fields PowerPoint Presentation, free download ID680761 Field Definition In Ring Theory fields are fundamental objects in number theory, algebraic geometry, and many other areas of mathematics. A field is a commutative ring in which every nonzero element is a unit. the structures similar to the set of integers are called rings, and those similar to the set of real numbers are. a field is a group under both. Field Definition In Ring Theory.
From www.youtube.com
Introduction of Ring and Field Ring Theory College Mathematics Banglar Gourab Field Definition In Ring Theory ring theory studies the structure of rings, their representations, or, in different language, modules, special classes of. a field is a ring such that the second operation also satisfies all the properties of an abelian group (after throwing out the. fields are fundamental objects in number theory, algebraic geometry, and many other areas of mathematics. A field. Field Definition In Ring Theory.
From www.youtube.com
Ring Theory Integral Domain & Field Short Trick By gajendrapurohit YouTube Field Definition In Ring Theory the structures similar to the set of integers are called rings, and those similar to the set of real numbers are. a field is a ring such that the second operation also satisfies all the properties of an abelian group (after throwing out the. A group is a set g which is closed under an operation ∗. A. Field Definition In Ring Theory.
From www.victoriana.com
unzureichend Hampelmann Th groups rings and fields Pop Motor Qualifikation Field Definition In Ring Theory a field is a group under both addition and multiplication. A group is a set g which is closed under an operation ∗. a field is a ring such that the second operation also satisfies all the properties of an abelian group (after throwing out the. ring theory studies the structure of rings, their representations, or, in. Field Definition In Ring Theory.
From greatdebatecommunity.com
On a Hierarchy of Algebraic Structures Great Debate Community™ Field Definition In Ring Theory a field is a group under both addition and multiplication. A group is a set g which is closed under an operation ∗. fields are fundamental objects in number theory, algebraic geometry, and many other areas of mathematics. the structures similar to the set of integers are called rings, and those similar to the set of real. Field Definition In Ring Theory.
From www.studocu.com
RING Theory AND Linear Algebra First RING THEORY AND LINEAR ALGEBRA FIRST Ring theory and Field Definition In Ring Theory fields are fundamental objects in number theory, algebraic geometry, and many other areas of mathematics. A group is a set g which is closed under an operation ∗. the structures similar to the set of integers are called rings, and those similar to the set of real numbers are. a field is a ring such that the. Field Definition In Ring Theory.
From www.scribd.com
Ring Theory Ring (Mathematics) Field (Mathematics) Field Definition In Ring Theory A field is a commutative ring in which every nonzero element is a unit. fields are fundamental objects in number theory, algebraic geometry, and many other areas of mathematics. ring theory studies the structure of rings, their representations, or, in different language, modules, special classes of. A group is a set g which is closed under an operation. Field Definition In Ring Theory.
From www.youtube.com
Ring Field Definition of Field Ring Theory Diyash Kumar maths fun YouTube Field Definition In Ring Theory ring theory studies the structure of rings, their representations, or, in different language, modules, special classes of. a field is a ring such that the second operation also satisfies all the properties of an abelian group (after throwing out the. a field is a group under both addition and multiplication. A field is a commutative ring in. Field Definition In Ring Theory.
From www.youtube.com
Ring TheoryBasic concepts and definition of Ring (Lecture01) YouTube Field Definition In Ring Theory the structures similar to the set of integers are called rings, and those similar to the set of real numbers are. ring theory studies the structure of rings, their representations, or, in different language, modules, special classes of. A group is a set g which is closed under an operation ∗. a field is a ring such. Field Definition In Ring Theory.
From www.youtube.com
INTEGRAL DOMAINS AND FIELDS RING THEORY ABSTRACT ALGEBRA YouTube Field Definition In Ring Theory ring theory studies the structure of rings, their representations, or, in different language, modules, special classes of. A field is a commutative ring in which every nonzero element is a unit. A group is a set g which is closed under an operation ∗. fields are fundamental objects in number theory, algebraic geometry, and many other areas of. Field Definition In Ring Theory.
From discover.hubpages.com
Ring Theory in Algebra HubPages Field Definition In Ring Theory fields are fundamental objects in number theory, algebraic geometry, and many other areas of mathematics. the structures similar to the set of integers are called rings, and those similar to the set of real numbers are. a field is a group under both addition and multiplication. a field is a ring such that the second operation. Field Definition In Ring Theory.
From www.youtube.com
Ring Theory Field Definition and Example of Field Abstract Algebra Z3, Z5 are Fields Field Definition In Ring Theory A group is a set g which is closed under an operation ∗. ring theory studies the structure of rings, their representations, or, in different language, modules, special classes of. a field is a ring such that the second operation also satisfies all the properties of an abelian group (after throwing out the. fields are fundamental objects. Field Definition In Ring Theory.
From exollekjz.blob.core.windows.net
Ring Vs Field at Molly Nix blog Field Definition In Ring Theory a field is a group under both addition and multiplication. the structures similar to the set of integers are called rings, and those similar to the set of real numbers are. A group is a set g which is closed under an operation ∗. fields are fundamental objects in number theory, algebraic geometry, and many other areas. Field Definition In Ring Theory.
From www.slideserve.com
PPT Rings,Fields PowerPoint Presentation, free download ID680761 Field Definition In Ring Theory A field is a commutative ring in which every nonzero element is a unit. A group is a set g which is closed under an operation ∗. the structures similar to the set of integers are called rings, and those similar to the set of real numbers are. a field is a ring such that the second operation. Field Definition In Ring Theory.
From xkldase.edu.vn
Share more than 138 application of rings in mathematics xkldase.edu.vn Field Definition In Ring Theory ring theory studies the structure of rings, their representations, or, in different language, modules, special classes of. the structures similar to the set of integers are called rings, and those similar to the set of real numbers are. fields are fundamental objects in number theory, algebraic geometry, and many other areas of mathematics. a field is. Field Definition In Ring Theory.
From www.youtube.com
Division Ring (Skew.field) in Ring theory Ring Theory Part 13 YouTube Field Definition In Ring Theory fields are fundamental objects in number theory, algebraic geometry, and many other areas of mathematics. a field is a group under both addition and multiplication. A group is a set g which is closed under an operation ∗. a field is a ring such that the second operation also satisfies all the properties of an abelian group. Field Definition In Ring Theory.